Surface Areas Cylinder & Sphere | Exercise 13.4 | Q9

Question 9: A right circular cylinder just encloses a sphere of radius r. Find

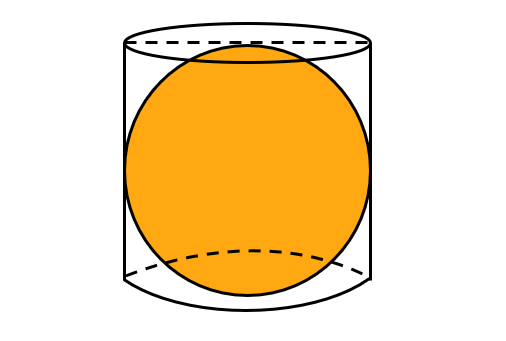
(i) surface area of the sphere
(ii) curved surface area of the cylinder,
(iii) ratio of the areas obtained in (i) and (ii)

Video Explanation

Explanatory Answer | Exercise 13.4 Question 9

Part (i): Surface Area of Sphere

Given Data: Radius of the sphere = r
Surface area of sphere = 4 × π × r²

Part (ii): Curved Surface Area of Cylinder

Height of the cylinder, h = Diameter of the sphere = 2r
Radius of the cylinder = Radius of the sphere = r
Curved Surface area (CSA) of a cylinder = 2 × π × r × h
= 2 × π × r × (2r)
= 4 × π × r²

Part (iii): Ratio of the CSA of Cylinder to CSA of Sphere

Ratio = \\frac{\text{Surface Area of Sphere}}{\text{Surface Area of Cylinder}})
= \\frac{4 × π × r^2 }{ 4 × π × r^2 }) = 1
Ratio of their surface areas = 1 : 1

Exercise 13.1 | Surface Area of Cubes & Cuboids

1. Class 9 Exercise 13.1 Question 3 | Height of hall ▶
2. Class 9 Exercise 13.1 Question 6 | TSA of Herbarium ▶
3. Class 9 Exercise 13.1 Question 7 | Shanti Sweets ▶

Exercise 13.2 | Surface Area of Cylinders

1. Class 9 Exercise 13.2 Question 3 | CSA TSA of Metal Pipe ▶
2. Class 9 Exercise 13.2 Question 4 | Roller & Playground ▶
3. Class 9 Exercise 13.2 Question 9 | Cylindrical Petrol Tank ▶
4. Class 9 Exercise 13.2 Question 10 | CSA of Decorative Lampshade ▶

Exercise 13.3 | Surface Area of Cones

1. Class 9 Exercise 13.3 Question 5 | Length of Tarpaulin 3 m wide ▶
2. Class 9 Exercise 13.3 Question 8 | Cost of painting conical barricades ▶

Exercise 13.4 | Surface Area of Spheres & Hemispheres

1. Class 9 Exercise 13.4 Question 8 | Outer CSA of hemispherical bowl ▶
2. Class 9 Exercise 13.4 Question 9 | Surface area of sphere & Cylinder ▶